Complex Numbers Operations Complex Conjugates And The Linear Factorization Theorem

Complex Numbers Operations Complex Conjugates And The Linear In getting through algebra, we never talked about complex numbers, but they are important so let's discuss them now! these are numbers with a real component. When complex numbers are viewed as points in the euclidean plane \(\mathbb{r}^{2}\), several of the operations defined in section 2.2 can be directly visualized as if they were operations on vectors. for the purposes of this chapter, we think of vectors as directed line segments that start at the origin and end at a specified point in the euclidean plane.

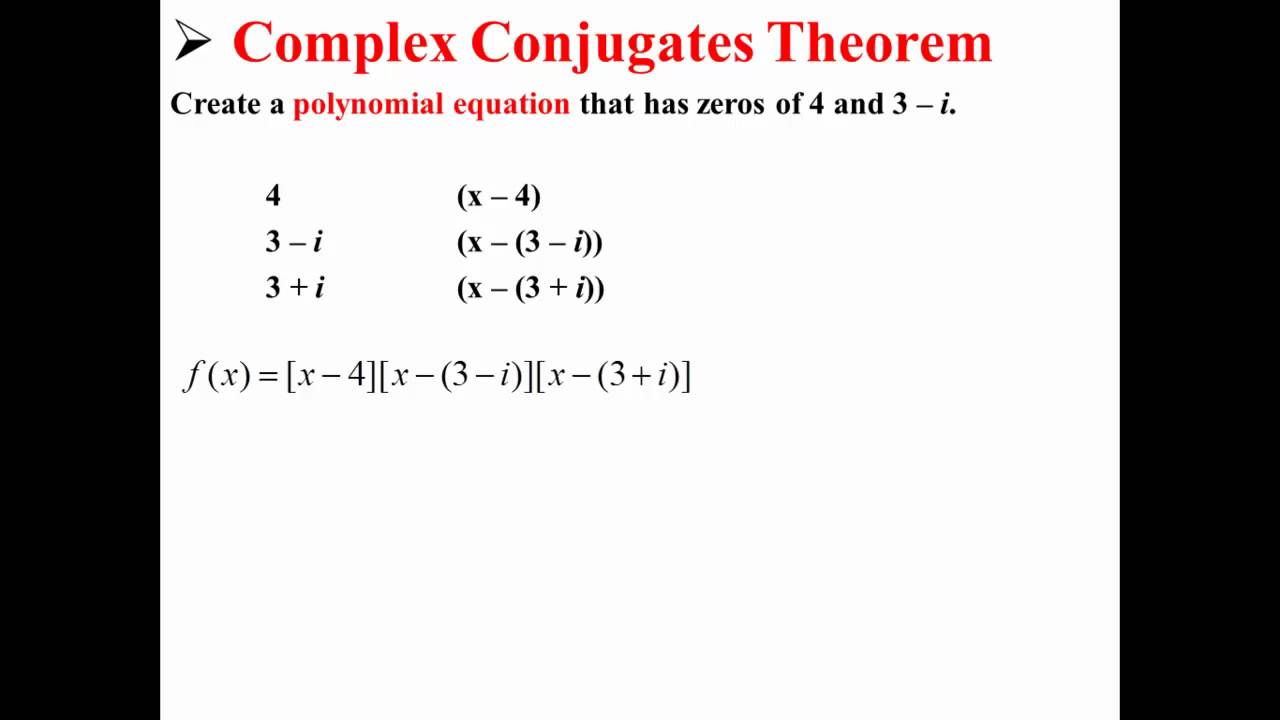
Complex Conjugates Theorem Youtube Addition and subtraction of complex numbers has the same geometric interpretation as for vectors. the same holds for scalar multiplication of a complex number by a real number. (the geometric interpretation of multiplication by a complex number is di erent; we’ll explain it soon.) complex conjugation re ects a complex number in the real axis. 2. The result of adding, subtracting, multiplying, and dividing complex numbers is a complex number. the product of complex conjugates, \(a bi\) and \(a − bi\), is a real number. use this fact to divide complex numbers. multiply the numerator and denominator of a fraction by the complex conjugate of the denominator and then simplify. Irreducible quadratic factors: if p is a polynomial with real coefficients, p can be factored into a product of linear factors (which are not necessarily distinct) and irreducible quadratic factors having real coefficients. complex conjugates: if p is a polynomial with real coefficients, complex zeros must occur in conjugate pairs. if. Definition 6.1.2: inverse of a complex number. let z = a bi be a complex number. then the multiplicative inverse of z, written z − 1 exists if and only if a2 b2 ≠ 0 and is given by. z − 1 = 1 a bi = 1 a bi × a − bi a − bi = a − bi a2 b2 = a a2 b2 − i b a2 b2. note that we may write z − 1 as 1 z.

Conjugates Of Complex Numbers Properties And Solved Examples Irreducible quadratic factors: if p is a polynomial with real coefficients, p can be factored into a product of linear factors (which are not necessarily distinct) and irreducible quadratic factors having real coefficients. complex conjugates: if p is a polynomial with real coefficients, complex zeros must occur in conjugate pairs. if. Definition 6.1.2: inverse of a complex number. let z = a bi be a complex number. then the multiplicative inverse of z, written z − 1 exists if and only if a2 b2 ≠ 0 and is given by. z − 1 = 1 a bi = 1 a bi × a − bi a − bi = a − bi a2 b2 = a a2 b2 − i b a2 b2. note that we may write z − 1 as 1 z. Plex number a biis the complex number a bi. geometrically, com plex conjugation re ects a complex number across the real axis. the complex conjugate of zis written z: a bi= a bi theorem. complex conjugation satis es: z = z; w z= w z; wz= wz: a complex number zis real exactly when z= zand is purely imaginary exactly when z = z. Complex numbers. a complex number is a number that can be written in the form a bi a bi, where a a and b b are real numbers and i i is the imaginary unit defined by i^2 = 1 i2 = −1. the set of complex numbers, denoted by \mathbb {c} c, includes the set of real numbers \left ( \mathbb {r} \right) (r) and the set of pure imaginary numbers.