Definition Of Cauchy Sequence Lopicap
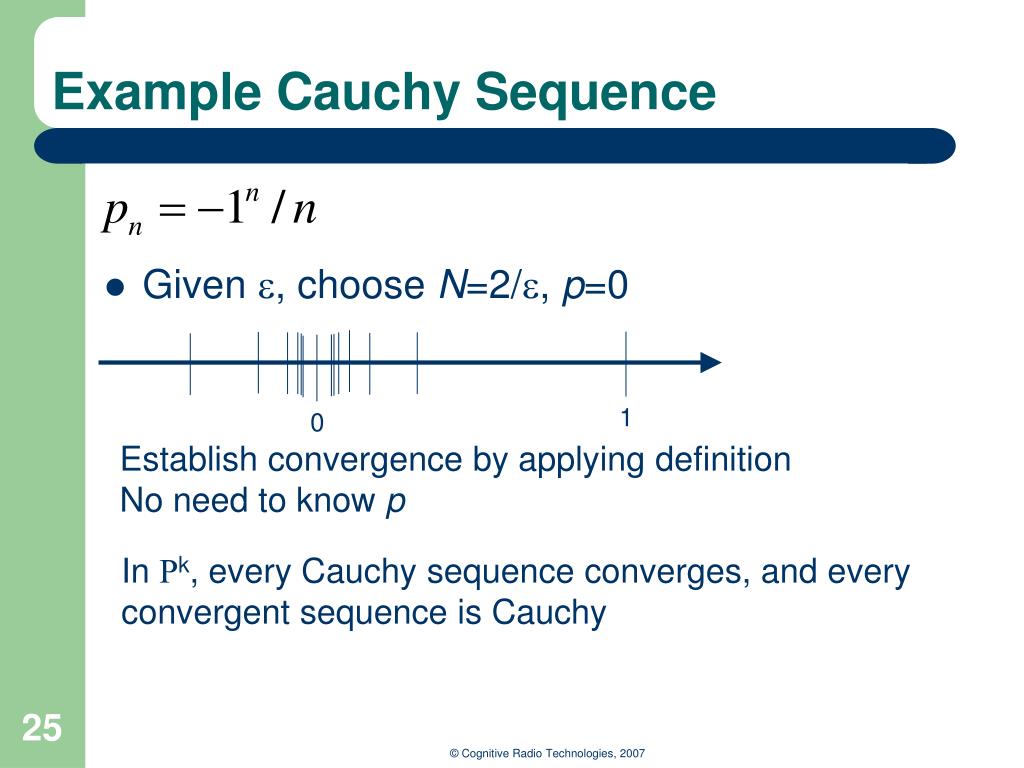
Definition Of Cauchy Sequence Lopicap Cauchy sequence. (a) the plot of a cauchy sequence shown in blue, as versus if the space containing the sequence is complete, then the sequence has a limit. (b) a sequence that is not cauchy. the elements of the sequence do not get arbitrarily close to each other as the sequence progresses. in mathematics, a cauchy sequence is a sequence whose. 3) perhaps: draw a straight line representing the real numbers, and an interval i: = (− ϵ, ϵ) of length epsilon> 0 around zero, thus: the existence of that n and the seq. being cauchy means that any pair of elements of the seq. with indexes greater than this number have a difference that is a number within i. share.

Famous Mathematicians Augustin Louis Cauchy The Father Of Analysis Cauchy sequences are useful because they give rise to the notion of a complete field, which is a field in which every cauchy sequence converges. because the cauchy sequences are the sequences whose terms grow close together, the fields where all cauchy sequences converge are the fields that are not ``missing" any numbers. the canonical complete. Using the definition of a cauchy sequence, prove that (1 n2) is a cauchy sequence. the utility of cauchy sequences lies in the fact that in a complete metric space, the criterion for convergence depends only on the terms of the sequence itself. more precisely, given any small positive distance, all but a finite number of elements of the. A cauchy sequence is a sequence of elements in a metric space where, for any given positive distance, there exists a point in the sequence beyond which all subsequent elements are within that distance of each other. this concept is crucial when discussing convergence, particularly in the context of completing spaces and understanding how sequences behave as they approach limits. Theorem 3.13.4. (cauchy's convergence criterion). a sequence {¯ xm} in en (*or cn ) converges if and only if it is a cauchy sequence. unfortunately, this theorem (along with the bolzano weierstrass theorem used in its proof) does not hold in all metric spaces. it even fails in some subspaces of e1.
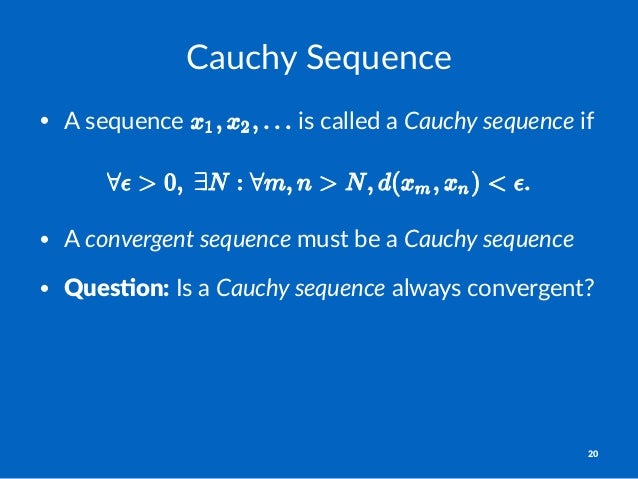
Definition Of Cauchy Sequence Lopicap A cauchy sequence is a sequence of elements in a metric space where, for any given positive distance, there exists a point in the sequence beyond which all subsequent elements are within that distance of each other. this concept is crucial when discussing convergence, particularly in the context of completing spaces and understanding how sequences behave as they approach limits. Theorem 3.13.4. (cauchy's convergence criterion). a sequence {¯ xm} in en (*or cn ) converges if and only if it is a cauchy sequence. unfortunately, this theorem (along with the bolzano weierstrass theorem used in its proof) does not hold in all metric spaces. it even fails in some subspaces of e1. Definition 1. a sequence {an} is said to be cauchy (or to be a cauchy sequence) if for every real number 0, there is an integer n (possibly depending on ) for which. theorem 2. a sequence of real numbers is convergent if and only if it is cauchy. proof. (⇒) let {an} be a convergent sequence with limit l. The really remarkable thing is that the converse is true: if {a n} n = 1 ∞ is a cauchy sequence of real numbers, then it must be convergent. the proof of this theorem, like bolzano weierstrass, is a bit involved. the first step is the following intermediate result, which is often useful in its own right. if {a n} n = 1 ∞ is cauchy, then it.

Ppt Limit Cycle Powerpoint Presentation Free Download Id 413160 Definition 1. a sequence {an} is said to be cauchy (or to be a cauchy sequence) if for every real number 0, there is an integer n (possibly depending on ) for which. theorem 2. a sequence of real numbers is convergent if and only if it is cauchy. proof. (⇒) let {an} be a convergent sequence with limit l. The really remarkable thing is that the converse is true: if {a n} n = 1 ∞ is a cauchy sequence of real numbers, then it must be convergent. the proof of this theorem, like bolzano weierstrass, is a bit involved. the first step is the following intermediate result, which is often useful in its own right. if {a n} n = 1 ∞ is cauchy, then it.

Comments are closed.