Integrating Exponential Functions

Ca Tf 5 Integrating Exponential Functions Youtube Integrals of exponential functions. exponential functions can be integrated using the following formulas. ∫ exdx = ex c ∫ axdx = ax lna c ∫ e x d x = e x c ∫ a x d x = a x ln a c. the nature of the antiderivative of ex e x makes it fairly easy to identify what to choose as u u. if only one e e exists, choose the exponent of e e as u u. The exponential function is perhaps the most efficient function in terms of the operations of calculus. the exponential function, y = ex, is its own derivative and its own integral. rule: integrals of exponential functions. exponential functions can be integrated using the following formulas. ∫exdx = ex c ∫axdx = ax lna c.
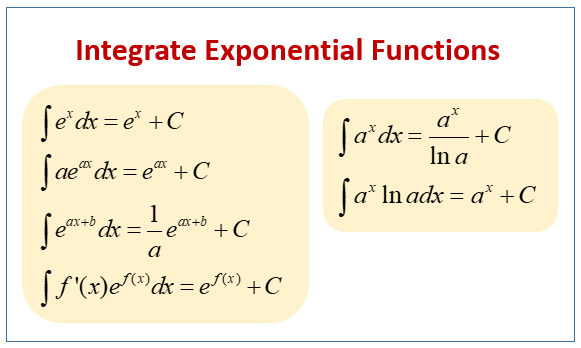
Integration Of Exponential And Natural Log Functions Examples Exponential and logarithmic functions are used to model population growth, cell growth, and financial growth, as well as depreciation, radioactive decay, and resource consumption, to name only a few applications. in this section, we explore integration involving exponential and logarithmic functions. integrals of exponential functions. Integration of exponential functions. exponential functions are those of the form f (x)=ce^ {x} f (x) = c ex for a constant c c, and the linear shifts, inverses, and quotients of such functions. exponential functions occur frequently in physical sciences, so it can be very helpful to be able to integrate them. Since the derivative of e^x is itself, the integral is simply e^x c. the integral of other exponential functions can be found similarly by knowing the properties of the derivative of e^x. Our most fundamental rule when integrating exponential functions are as follows: ∫ e x x d x = e x c ∫ a x x d x = a x ln. . a c. understanding ∫ e x x d x = e x c. the derivative of the exponential function, e x, is simply e x itself. we can apply the fundamental theorem of calculus to confirm the integral rule for e x.

In3 3 Integration Of Exponential Functions Learning Lab Since the derivative of e^x is itself, the integral is simply e^x c. the integral of other exponential functions can be found similarly by knowing the properties of the derivative of e^x. Our most fundamental rule when integrating exponential functions are as follows: ∫ e x x d x = e x c ∫ a x x d x = a x ln. . a c. understanding ∫ e x x d x = e x c. the derivative of the exponential function, e x, is simply e x itself. we can apply the fundamental theorem of calculus to confirm the integral rule for e x. Prove properties of logarithms and exponential functions using integrals. express general logarithmic and exponential functions in terms of natural logarithms and exponentials. we already examined exponential functions and logarithms in earlier chapters. however, we glossed over some key details in the previous discussions. Well, to find the antiderivative (integral) of an exponential function, we will apply the same three steps, except instead of multiply, we will divide! ∫ a b x d x = a b x b (ln a) c. a: the base of the exponential function. b: the constant that multiplies the variable ‘x’ in the exponent. this coefficient determines the growth or decay.

Integrating Exponential Functions By Substitution Antiderivatives Prove properties of logarithms and exponential functions using integrals. express general logarithmic and exponential functions in terms of natural logarithms and exponentials. we already examined exponential functions and logarithms in earlier chapters. however, we glossed over some key details in the previous discussions. Well, to find the antiderivative (integral) of an exponential function, we will apply the same three steps, except instead of multiply, we will divide! ∫ a b x d x = a b x b (ln a) c. a: the base of the exponential function. b: the constant that multiplies the variable ‘x’ in the exponent. this coefficient determines the growth or decay.
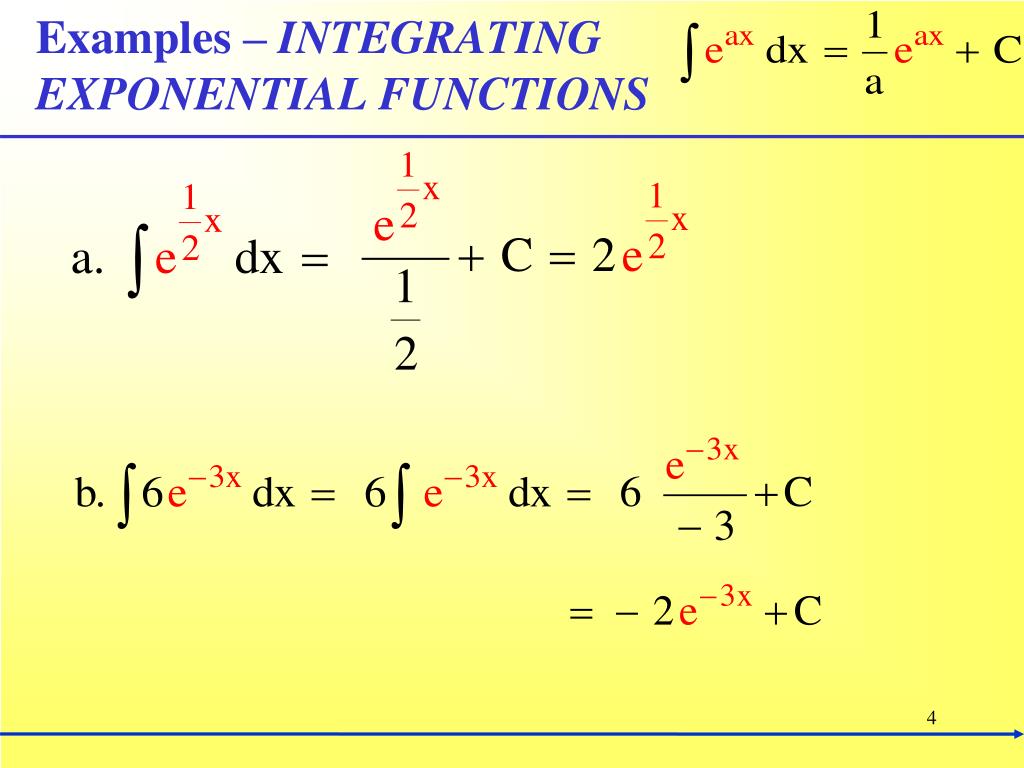
Ppt в 4 2 Integration Using Logarithmic And Exponential Functions

Comments are closed.