Linear Diophantine Equations With 3 Variables 3 Different Methods

Linear Diophantine Equations With 3 Variables 3 Different Methods We want to solve the linear diophantine equation with 3 variables:35x 55y 77z=1for integer solutions in three methods are discussed:1. we want to solve the linear diophantine equation with 3. Then substitute the expression for z into the equation that defines x ((3 10m 5z) 6) and simplify. you should get 5k 5m 12. these are your parameterized solutions to the general equation: (x, y, z) = (5k 5m 12, 6k 3m 10, 15 4m 6k), where k and m represent any positive or negative integer.

How To Solve A Linear Diophantine Equation With Pictures A linear diophantine equation (lde) is an equation with 2 or more integer unknowns and the integer unknowns are each to at most degree of 1. linear diophantine equation in two variables takes the form of \(ax by=c,\) where \(x, y \in \mathbb{z}\) and a, b, c are integer constants. x and y are unknown variables. Equations with more than 2 variables. now, consider the linear diophantine equation in three variables ax by cz = d. ax by cz = d. again by bézout's identity, as a a and b b range over all integer values, the set of values ax by ax by is equal to the set of multiples of \gcd (a,b). gcd(a,b). In this video we examine two methods to solve a linear diophantine equation in three variables. the first method is a general method which works with all of. I’ll refer to diophantine equations, meaning equations which are to be solved over the integers. for example, the equation x3 y3 = z3 has many solutions over the reals. here’s a solution: x= 1, ,y= 1, z= 3 √ 2. however, this equation has no nonzero integer solutions. this is a special case of fermat’s last theorem.

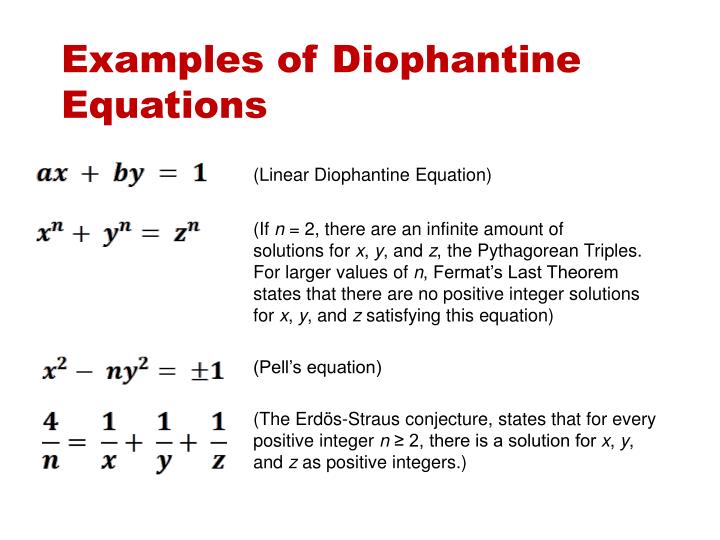
Ppt Diophantine Equations Powerpoint Presentation Id 2635081 In this video we examine two methods to solve a linear diophantine equation in three variables. the first method is a general method which works with all of. I’ll refer to diophantine equations, meaning equations which are to be solved over the integers. for example, the equation x3 y3 = z3 has many solutions over the reals. here’s a solution: x= 1, ,y= 1, z= 3 √ 2. however, this equation has no nonzero integer solutions. this is a special case of fermat’s last theorem. The simpler class of linear diophantine equations. solving a linear equation in one variable over the integers is trivial (the solution to ax = b is x = b=a, assuming a is nonzero and divides b). so the simplest interesting equations are linear equations in two variables. the general form of a linear equation in two variables is. Theorem 8.3.1. let a, b, and c be integers with a ≠ 0 and b ≠ 0.if a and b are relatively prime, then the linear diophantine equation ax by = c has infinitely many solutions. in addition, if x0, y0 is a particular solution of this equation, then all the solutions of the equation are given by. x = x0 bk y = y0 − ak.

Cm 3 Simple Linear Diophantine Equations Simple Linear Diophantineо The simpler class of linear diophantine equations. solving a linear equation in one variable over the integers is trivial (the solution to ax = b is x = b=a, assuming a is nonzero and divides b). so the simplest interesting equations are linear equations in two variables. the general form of a linear equation in two variables is. Theorem 8.3.1. let a, b, and c be integers with a ≠ 0 and b ≠ 0.if a and b are relatively prime, then the linear diophantine equation ax by = c has infinitely many solutions. in addition, if x0, y0 is a particular solution of this equation, then all the solutions of the equation are given by. x = x0 bk y = y0 − ak.